Editorial
, Volume: 6( 2)Time-Frame Conversion
Received: April 26, 2018; Accepted: May 30, 2018; Published: June 6, 2018
Citation: Főfai S. Time-Frame Conversion. J Phys Astron. 2018; 6(2):151
Abstract
https://sporbahisleri.blogaaja.fi http://sporbahisleri.parsiblog.com https://spor-bahisleri.jimdosite.com https://sporbahisleri.edublogs.org https://sporbahisleri.websites.co.in https://sporbahisleri.podia.com https://sporbahisleri7.wordpress.com https://sporbahisleri.jigsy.com https://niwn-chroiaty-mcieung.yolasite.com https://spor-bahisleri.mywebselfsite.net https://sporbahisleri.mystrikingly.com https://sporbahisleri.splashthat.com https://sporbahisleri1.webnode.com.tr https://sporbahisleri.odoo.com http://sporbahisleri.creatorlink.net http://www.geocities.ws/sporbahisleri/ https://spor-s-site.thinkific.com https://artistecard.com/sporbahisleri https://sporbahisleri.estranky.cz https://spor-bahisleri.mozellosite.com https://651be6b563e56.site123.me https://betsitesiinceleme.blogspot.com https://sporbahisleri.hashnode.dev https://sporbahislerim.wixsite.com/spor-bahisleri https://sporbahislerix.weebly.com https://sites.google.com/view/betsiteleri https://codepen.io/sporbahisleri https://sporbahisleri.bcz.com https://www.smore.com/6rsb9
Introduction
About Time-frame Conversion
When an Observer measures the time of events of another Source, the time needed of the transferring media to travel the distances between the Source and Observer will distort the perceived time when they move relative to each other. Doppler Effect determines the frequencies using the angle of movement and velocity, but not calculating the exact changes of distance during the observed period, so its results are correct only in special circumstances. Special Relativity didn’t take into consideration the distances either, however its correction near right angle in special cases can produce closer results to the exact values, than Doppler Effect. Below I’d like to show the relation between the real time and perceived time of events and present a simple method of calculating them on the base of changing distance.
I call Time-frame the time length of examined events of Source (Q) and I distinguish the real Time-frame (Tf) of events and perceived Time-frame (Tf’) that is the time period of events measured by the Observer (O). Calculating the exact Time frames of events is integrant to determine the real time length of the perceived phenomena, or calculating the real frequencies of Sources dividing the number of impulses by it.
The base hypothesis of Time-frame Conversion is that the speed of the media (light) transferring the impulses is constant in space, independently from the Source or Observer. The key element of Time-frame conversion is the determination of distance. The more precise the change of distance is determined, the more precise the conversion.
Time-frame Conversion
With the help of time, we can measure the sequence of events or impulses, or frequency (f). I call “real time (t)” the appearance of an impulse of the Source (Q), and “perceived time (t’)” the time when the event reaches the Observer (O): t’= t + d/c
Let’s call “real Time-frame (Tf = t1 - t0)” the time elapsed between two impulses of Source, and “perceived Time-frame (Tf’=t’1-t’0)” the period perceived by Observer.
When an impulse from Source reaches the Observer with a speed of light (c), we can calculate the perceived Time-frame by knowing this speed and the change of distance (Δd= d - d’) from Source, where d=distance covered by first impulse, d’= distance covered by last impulse:
Tf’= Tf + Δd/c This is the basic equation of Time-frame conversion
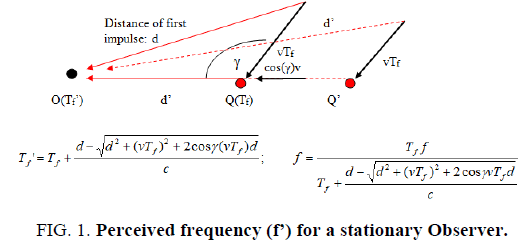
Knowing the distance (d), the angle (γ) and the speed (v) of the Source (Q) and its Time-frame we can calculate the perceived frequency (f’) for a stationary Observer as follows (there are many more method) FIG. 1.
Figure 1: Perceived frequency (f’) for a stationary Observer.
It can be seen that converting Time-frame we must know beyond the angle of movement and the speed of source its distance, and the measured length of period, because all of these factors can modify Δd.
It can be shown that when γ= 0° or 180° (cos γ= 1;-1) then Time-frame conversion equals with Doppler effect, and when γ= 90° (cos γ= 0) and d/c= Tf then Time-frame conversion gives the result of Special Relativity (Lorentz factor), so in some point of view Time-frame conversion unifies the two methods, while showing their deficiencies as well. (that is Doppler effect gives only average values and Special Relativity can be used only in very special cases).
All impulses travelling the same route will reach the Observer during the same time, so when the distance doesn’t change (d1= d0→ Δd= 0) then Tf’= Tf and f’= f which means that gravitation cannot modify the perceived Time-frame (it would cause frequency jam, so gravitation alone can modify the speed of light or energy of electromagnetic waves but not their frequencies, that is their Time-frames).
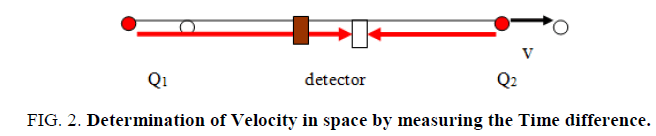
Contrasting Special Relativity the moving ‘Inertia-systems’ are not equals, their relative movements can be determined provided the transferring media (e.g. light) isn’t dragged by them. The Doppler effect of starlight proves this, where light travels with constant speed, and Doppler effect is caused by the relative speed of Source and Observer to it FIG. 2.
Figure 2: Determination of Velocity in space by measuring the Time difference.
E.g when two sources (Q1, Q2) are travelling in space with a detector between them their velocity (v) in space can be determined by measuring the time difference between the arriving signs from them:
(In Michelson – Morley experiment the drag of ‘ether’ wasn’t eliminated, which phenomena was proved by Fizeau in 1851. A new experiment is needed in outer space, with modern devices, where light beams aren’t slowed down by any material. It would be useful to examine the effect of gravitation on the speed of light too).
Examples of identities (Doppler Effect and SR):
When Source moves in line with Observer the factor of Time-frame conversion equals with that of Doppler Effect, because cosine 0°=1 and 180°=-1 so Δd depends alone on the velocity.
Example of a simple calculation when Source emits 2 impulses in Tf period moving toward Observer. Observer perceives the event in Tf’ period FIG. 3. This case we get the factor of Doppler Effect: (1-v/c)
Figure 3: Source moves in line with Observer.
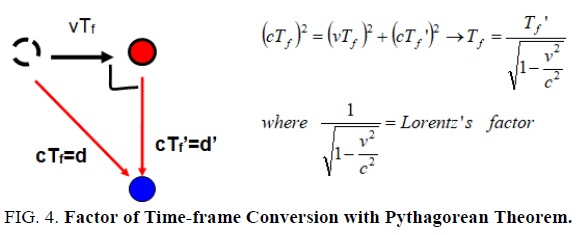
When a right angle can be drawn with the relative movement of Source and Observer and with the first and last impulse, we can determine the factor of Time-frame Conversion with Pythagorean Theorem FIG.4. This case we get Lorentz’s factor, used in Special Relativity:
Figure 4: Factor of Time-frame Conversion with Pythagorean Theorem.
There can be several method and equation to convert Time-frames, when the movements are more complicated in space, the main thing is to determine the change of distances the most precise way.
