Original Article
, Volume: 11( 6)Structure Simulation and Study of Electronic and Dielectric Properties of Unfluorinated and Fluorinated 1,4 Lactone
- *Correspondence:
- Sreepad HR , Research Centre, P.G. Department of Physics, Government College (Autonomous), Affiliated to University of Mysore, Mandya-571401, Karnataka, India, Tel: +91-9449184109, E-mail: hrsreepad@gmail.com
Received: August 31, 2016; Accepted: September 12, 2016; Published: September 15, 2016
Citation: Kirankumar BM, Nisarga KS, Neethashree DB, et al. Structure Simulation and Study of Electronic and Dielectric Properties of Unfluorinated and Fluorinated 1,4 Lactone. Chem Technol Ind J. 2016;11(6):103.
Abstract
First-principles calculations based on density functional theory have been done on the technologically important organic material 2,3-dideoxy-d-erythro-hex-2-enono-1,4-lactone [C6H8O4]. The triclinic structure of the material has been simulated and the structural parameters are found to be a=4.057Å, b=4.263Å, c=8.261Å, α=88.62°, β=80.36°, γ=65.13°. After fluorination the structural parameters are found to be a=4.156Å, b=4.488Å, c=7.652Å, α=83.10°, β=84.51°, γ=84.82°. Electron density of states (EDOS) has been computed in the material using the electronic structure calculation code of quantum-espresso which gives a band gap of 3.17 eV. After fluorination the band gap is found to be 3.14 eV. The value of dielectric constant in the material comes out to be 2.2508, 2.4991 and 2.9935 along x, y and z axes respectively and the average value comes out to be 2.58. After fluorination the dielectric constant of the compound comes out to be 2.3631, 2.3547 and 2.7103 along x, y and z axes respectively and the average value comes out to be 2.48. The computed phonon modes range from 357 cm-1 to 3555 cm-1. After fluorination the phonon modes range from 300 cm-1 to 3493 cm-1.
Keywords
1,4-Lactone; First-principles calculation; Electron density of states (EDOS); Band gap; Dielectric constant; Phonon modes
Introduction
Lactones have attracted the scientific community owing to their potential biological applications [1]. Okamura [2] have found L-gulono-1,4-lactone oxidase activity in Grifola frondosa. Keates et al. [3] have found that extracts of sclerotia from Sclerotinia sclerotiorum, a fungal phytopathogen, contain two electrochemically active constituents, d-glycero-pent-2-enono-1,4-lactone and 5-O-(α-d-galactopyranosyl)-d-glycero-pent-2-enono-1,4-lactone. Robinson et al. [4] have extracted anticancer sesquiterpene lactone from the roots of Saussurea lappa.
Huh et al. [5] have characterized D-arabinono-1,4-lactone oxidase obtained from Candida albicans ATCC 10231. Nishikimi et al. [6] have studied the cloning and chromosomal mapping of the human nonfunctional gene for L-gulono-gamma-lactone oxidase, the enzyme for L-ascorbic acid biosynthesis which is missing in man. Hervas et al. [7] have studied the communication between L-galactonon-1,4-lactone dehydrogenase and cytochrome c.
Ostergaard et al. [8] have carried out isolation of a cDNA coding for L-galactono-gamma-lactone dehydrogenase, an enzyme involved in the biosynthesis of ascorbic acid in plants. Imai et al. [9] have extracted L-galactono-gamma-lactone dehydrogenase from mitochondria of sweet potato tuberous roots. Schertl et al. [10] have used L-galactono-1,4-lactone dehydrogenase (GLDH) as a catalyst in the terminal step of the Smirnoff-Wheeler pathway for vitamin C (L-ascorbate) biosynthesis in plants. Fun et al. [11] have studied the structure of 2,3-dideoxy-d-erythro-hex-2-enono-1,4-lactone [1,4-lactone (C6H8O4)] using XRD.
It has been found that any little modification in the structure and composition of a material will bring in sufficient changes in the properties of the material [12,13]. Thus it is important to study the structure of the materials and look at the parameters which can be altered to get a better material for technological applications. First-principles calculation based on density functional theory [14] has been proved to be an effective tool in the study of structural, electronic and dielectric properties of organic materials [15,16]. With this in view, structure of 1,4 Lactone (C6H8O4) has been simulated using first-principles calculations based on density functional theory and computation of electronic density of states, dielectric constant and phonon modes have been done and the results have been reported in the present paper.
Computational Details
Several codes are available for the theoretical structure simulation [17]. The density functional theory approach has emerged as a well-established computational method. It has been widely employed to arrive at the conformations of a large number of molecular systems. The practical applicability and sophistication of DFT is strongly sensitive to the good choice of exchange-correlation function along with the appropriate basis set.
Quantum espresso is an integrated suite of open-source computer codes for electronic-structure calculations and materials modelling. It is based on density-functional theory, plane waves, and pseudopotentials. Authors have used plane wave self-consistent field (PWSCF) [18] implementation of density functional theory (DFT), with a local density approximation (LDA) [19] to exchange correlation energy of electrons and ultra-soft pseudopotentials [20], to represent interaction between ionic cores and valence electrons. Kohn-Sham wave functions were represented with a plane wave basis with an energy cutoff of 30 Ry and charge density cutoff of 180 Ry. Integration over brillouin zone was sampled with a Monkhorst-Pack scheme [21] with appropriate k point mesh and occupation numbers were smeared using Methfessel-Paxton scheme [22] with broadening of 0.03 Ry. The structure was relaxed to minimize energy.
Results and Discussion
In the present study, the triclinic unit cell of 1,4 Lactone was first simulated using “Avogadro” [23]. Later, atomic positions of the molecules have been used in the plane wave self-consistent field calculations. The structure was relaxed and the optimized values of the unit cell parameters thus arrived at through minimization of energy are; a=4.057Å, b=4.263Å, c=8.261Å, α=88.62°, β=80.36°, γ=65.13°. “scf'” calculation was done using the final atomic positions obtained after relaxing the structure using the program 'pw.x' of quantum espresso. Fluorination of 1,4 Lactone was done by replacing one hydrogen atom by fluorine atom. Again the structure was relaxed to minimize the energy and the lattice parameters thus arrived are; a=4.156Å, b=4.488Å, c=7.652Å, α=83.10°, β=84.51°, γ=84.82°.
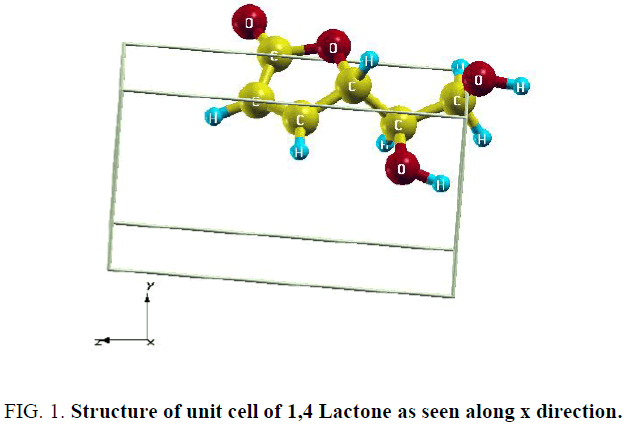
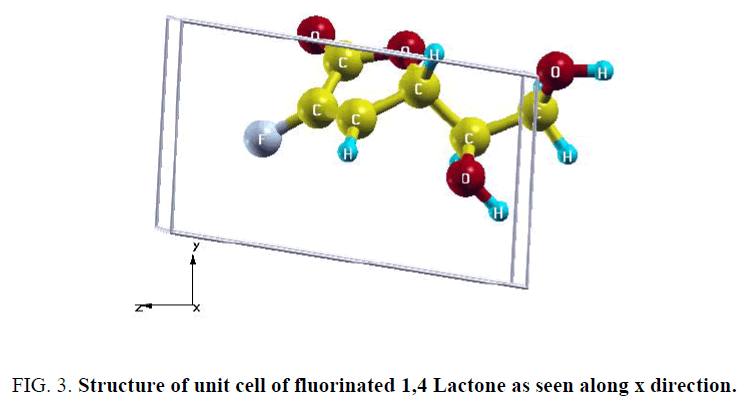
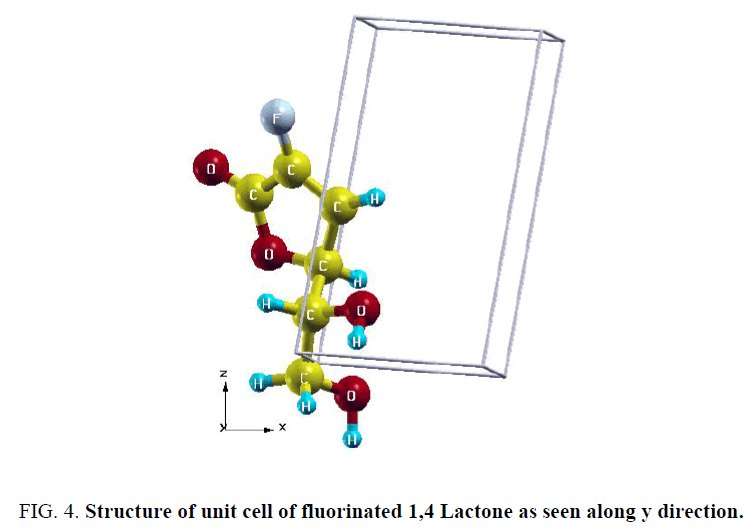
The completely relaxed structure of the unit cell was visualized using the program “XCrysDen” [24] and the structure of unit cell of 1,4 Lactone as seen along X-axis and Y-axis are shown in ( Figure 1)-( 4). The bond lengths and bond angles in the relaxed structure of 1,4 Lactone have been tabulated in Tables 1 and 2 respectively.
| Bond | Bond length (Å) |
|---|---|
| C–H | 1.11 |
| O–H | 1.00 |
| C–O | 1.24-1.43 |
| C–C | 1.37-1.50 |
Table 1: Bond length in 1,4 Lactone.
| Bond | Bond angle (deg) |
|---|---|
| O–C–O | 116 |
| H–C–H | 107 |
| H–C–C | 115, 118 |
| C–O–H | 110 |
| C–C–O | 111, 112 |
| O–C–H | 108, 111 |
| C-C-C | 112 |
Table 2: Bond angle in 1,4 Lactone.
EDOS Calculation
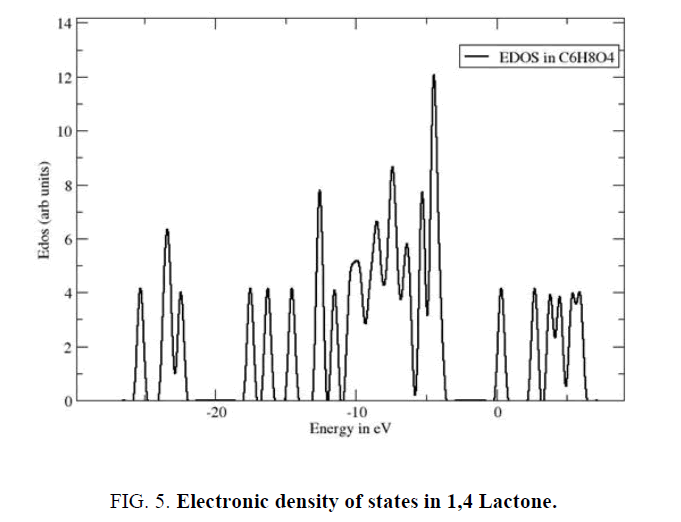
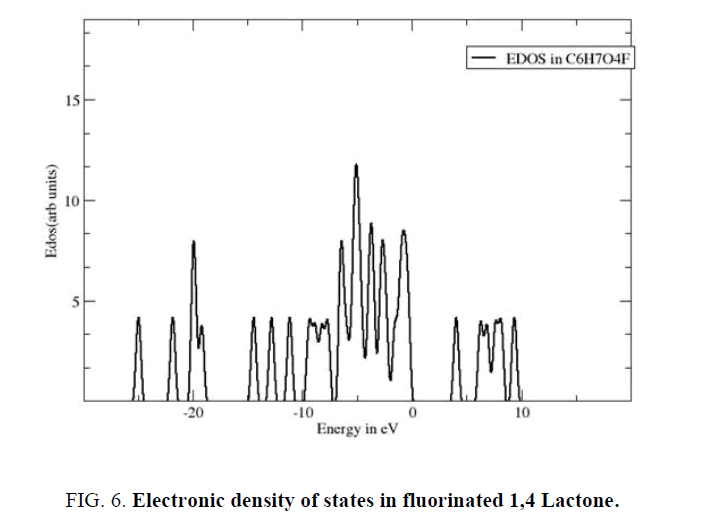
Electron density of states (EDOS) has been computed in 1,4 Lactone using electronic structure calculation code of quantum espresso. EDOS in 1,4 Lactone and fluorinated 1,4 Lactone have been shown in ( Figure 5) and ( 6). Band gap in 1,4 Lactone and fluorinated 1,4 Lactone is found to be 3.17 eV and 3.14 eV respectively. This value is close to that exhibited by non-linear optical (NLO) materials [25] and organic semiconducting materials.
Presently, the organic semiconducting materials have very much attracted the scientific community owing to their technological advantages over traditional silicon-based semiconductor devices. Most organic materials are much less expensive to generate than highly crystalline inorganic semiconductors, and also may be used to make devices with inexpensive fabrication methods. Also, most organic materials are soluble in one or more common solvents which allows for the possibility of solution processing which can produce many devices at very low cost [26-28].
Several inorganic NLO materials show a band gap in the range 2 eV to 4 eV. For example, lithium niobate shows a band gap of 4 eV. Barium titanate shows a value of 3.2 eV. BSO crystals show a value of 4.02 eV and KTN nanoparticles show a band gap of 3.2 6eV. The organic NLO material L-Tartaric acid shows an optical band gap of 3.65 eV. Tuning of the band gap plays an important role in the field of photonic crystals.
Dielectric constant and phonon modes
The dielectric constant and phonon modes of the material have been computed in unfluorinated and fluorinated state using the ph.x code of quantum espresso. Dielectric constant of the material has been computed. The value of dielectric constant in 1,4 Lactone comes out to be 2.2508, 2.4991 and 2.9935 along x, y and z axes respectively and the average value comes out to be 2.58. After fluorination the dielectric constant of the compound comes out to be 2.3631, 2.3547 and 2.7103 along x, y and z axes respectively the average value comes to be 2.48.
This value is in the range exhibited by low k dielectric materials used in the semiconductor industry. For example; dielectric constant of fluropolyimide is 2.8, benzo-cyclo-butane is 2.7, black diamond is 2.7, polyethylene is 2.4, polypropylene is 2.3, fluoropolymer is 2.24, perylene is 2.2 and in dupont PTFE-based copolymer AF 2400 it is 2.06. They have applications in the fabrication of semiconducting devices [29]. This also shows that the material under study can be used in semiconductor devices.
Phonon modes have been computed using the ph.x program of quantum espresso. The computed phonon modes range from 357 cm-1 to 3555 cm-1. After fluorination the phonon modes range from 300 cm-1 to 3493 cm-1.
All the phonon modes are positive and hence they clearly prove that the simulated structures are stable. When one hydrogen atom has been replaced by a fluorine atom which is heavier, the frequency range of phonons has come down in the material. Values of various parameters in unfluorinated and fluorinated 1,4 Lactone are tabulated in Table 3.
| Parameter | Unfluorinated1,4 Lactone | Fluorinated1,4 Lactone |
|---|---|---|
| a (Å) | 4.057 | 4.156 |
| b (Å) | 4.263 | 4.488 |
| c (Å) | 8.261 | 7.652 |
| α (deg) | 88.62 | 83.10 |
| β (deg) | 80.36 | 84.51 |
| γ (deg) | 65.13 | 84.80 |
| Crystal system | Triclinic | Triclinic |
| Band gap (eV) | 3.17 | 3.14 |
| Average Dielectric constant | 2.58 | 2.48 |
| Phonon modes (cm-1) | 357-3555 | 300-3493 |
Table 3: Different parameters in unfluorinated and fluorinated 1,4 Lactone.
Conclusions
The lattice parameters arrived at by the structural optimization using the first-principles calculations are a=4.057Å, b=4.263Å, c=8.261Å, α=88.62°, β=80.36°, γ=65.13°. After flourination lattice parameters are found to be a=4.156Å, b=4.488Å, c=7.652Å, α=83.10°, β=84.51°, γ=84.81°. The lattice parameters of the present study are matching well with the experimentally found values in the material. The material shows a band gap of 3.17 eV. After fluorination the band gap of the material reduces to 3.14 eV. These values of band gap tell that this material is behaving as an organic semiconductor. The material shows an average dielectric constant of 2.58 and after fluorination the average dielectric constant is reduced to 2.48. The computed phonon modes in the material range from 357 cm-1 to 3555 cm-1. After fluorination the phonon modes range from 300 cm-1 to 3493 cm-1. Thus it is demanding for further investigations in the material to explore the applications of this material in organic semiconductor electronics and NLO applications. Present study of electronic and dielectric properties of the material is clearly indicating that this material is having the band gap and dielectric constant exhibited by organic semiconducting materials and NLO materials. Also, the present study clearly reveals that the band gap of this material can be tuned by the fluorination.
Acknowledgments
Author thanks the Department of Collegiate Education, Government of Karnataka for permitting to carry out the research work. Also, author acknowledges the necessary facilities provided by the Government College (Autonomous), Mandya (Affiliated to University of Mysore), India.
References
- Albertsson AC, Varma IK. Recent Developments in Ring Opening Polymerization of Lactones for Biomedical Applications. Biomacromolecules. 2003;4(6):1466-86.
- Okamura M. Purification and properties of L-gulono-1,4-lactone oxidase from Grifola frondosa. J Nutr Sci Vitaminol. 2001;47(3):258-62.
- Keates SE, Loewus FA, Helms GL, et al. 5-O-(α-D-galactopyranosyl)-d-glycero-pent-2-enono-1,4-lactone: Characterization in the oxalate-producing fungus, Sclerotinia sclerotiorum. Phytochemistry. 1998;49(8):2397-2401.
- Robinson A, Kumar TV, Sreedhar E, et al. A new sesquiterpene lactone from the roots of Saussurea lappa: structure-anticancer activity study. BioorgMedChemLett. 2008;18(14):4015-7.
- Huh WK, Kim ST, Yang KS, et al. Characterisation of D-arabinono-1,4-lactone oxidase from Candida albicans ATCC 10231. Eur J Biochem. 1994;225(3):1073-9.
- Nishikimi M, Fukuyama R, Minoshima S, et al. Cloning and Chromosomal Mapping of the Human Nonfunctional Gene for L-Gulono-y-lactone Oxidase, the Enzyme for L-Ascorbic Acid Biosynthesis Missing in Man. J Biol Chem. 1994;269(18):13685-8.
- Hervas M, Bashir Q, Leferink NG, et al. Communication between (L)-galactono-1,4-lactone dehydrogenase and cytochrome c. FEBS J. 2013;280(8):1830-40.
- Ostergaard J, Persiau G, Davey MW, et al. Isolation of a cDNA coding for L-galactono-γ-lactone dehydrogenase, an enzyme involved in the biosynthesis of ascorbic acid in plants. J Biol Chem. 1997;272(48):30009-16.
- Imai T, Karita S, Shiratori G, et al. L-galactono-gamma-lactone dehydrogenase from sweet potato: purification and cDNA sequence analysis. Plant Cell Physiol. 1998;39(12):1350-8.
- Schertl P, Sunderhaus S, Klodmann J, et al. l-Galactono-1,4-lactone dehydrogenase (GLDH) Forms Part of Three Subcomplexes of Mitochondrial Complex I in Arabidopsis thaliana. J Biol Chem. 2012;287:14412-9.
- Fun HK, Shivakumar K, Ang HB, et al. 2,3-Dideoxy-D-erythro-hex-2-enono-1,5-lactone and 1,4 Lactone Obtained from Tri-O-acetyl-D-glucal. Acta Cryst. 1995;C51:1330-3.
- Sreepad HR, Hembram KPSS, Waghmare UV. First?principles Study of Electronic and Dielectric Properties of Polyoxymethylene. AIP Conf Proc.2011;1349:871-872.
- Sreepad HR. Structure simulation and study of electronic and dielectric properties of two derivatives of benzamide. Mol Crys Liq Cryst.2016;625(1):195-201.
- Payne MC, Teter MP, Allan DC, et al. Iterative minimization techniques for ab initio total-energy calculations: molecular dynamics and conjugate gradients. Rev Mod Phys. 1992;64(4):1045-97.
- Sreepad HR, Ravi HR, Ahmed K, Radiation induced changes in electronic and dielectric properties of polyoxymethylene. AIP Conf Proc. 2013;1447(1):793-4.
- Sreepad HR, Ramegowda M, Ahmed K, et al. First-principles study of structure and electronic properties of phenyl imidazole. AIP Conf Proc. 2013;1512:802-3.
- http://en.wikipedia.org/wiki/Molecular_modelling
- http://www.pwscf.org
- Perdew JP, Zunger A. Self-interaction correction to density-functional approximations for many-electron systems. Phys Rev B.1981;23:5048-79.
- Vanderbilt D. Soft self-consistent pseudopotentials in a generalized eigenvalue formalism. Phys Rev B.1990;41:7892-5.
- Monkhorst HJ, Pack JD. Soft self-consistent pseudopotentials in a generalized eigenvalue formalism. Phys Rev B. 1976;13:5188-92.
- Methfessel M, Paxton AT. High-precision sampling for Brillouin-zone integration in metals. Phys Rev B. 1989;40:3616-21.
- http://avogadro.openmolecules.net/wiki/
- Kokalj A. Computer graphics and graphical user interfaces as tools in simulations of matter at the atomic scale. Comp Mater Sci. 2003;28:155-68.
- Sreepad HR. First-principles study of L-Alanine. Int JRes Pharma Biomed Sci. 2012;3(2):797-801.
- Hoppe H, Sariciftci NS.Organic solar cells: An overview. JMaterRes.2004;19(7):1924-45.
- Brabec CJ, Hauch JA, Schilinsky P, et al.Production Aspects of Organic Photovoltaics and Their Impact on the Commercialization of Devices. MRS Bull.2005;30(1):50-2.
- Janata J, Josowicz M.Conducting polymers in electronic chemical sensors. Nature Materials. 2003;2:19-24.
- Singh R, Ulrich RK. High and low dielectric constant materials. ElectrochemSocInterface. 1999;8:26-30.