Original Article
, Volume: 11( 5) DOI: 10.37532/2320-6756.2023.11 (5).345Redefining Black Hole Entropy Due to Proportionality to the Fine Structure Constant
Received date: 12-May-2023, Manuscript No. tspa-23-98430; Editor assigned: 14-May-2023, Pre-QC No. tspa-23-98430 (PQ); Reviewed: 22- May-2023, QC No. tspa-23-98430 (Q); Revised: 25-May-2023, Manuscript No. tspa-23-98430 (R); Published: 27-May-2023, DOI. 10.37532/2320-6756.2023.11 (5).345
Citation: Huckelby K. Redefining Black Hole Entropy Due to Proportionality to the Fine Structure Constant. J. Phys. Astron.2023;11(5):345.
Abstract
In this article we propose a new definition of the entropy of black holes. For this we base ourselves on a reformulation of the HawkingBekenstein formula in such a way that, on the one hand, by introducing a new variable that represents the number of microstates and On the other hand, taking into account the mass of the black hole, we can conclude a new equation for the entropy of black holes.
Keywords
Black hole; Entropy; Microstates; Black hole mass
Introduction
In this paper I will present an equation which will show how the black hole entropy is proportional to the fine structure constant. Afterwards I will explain how this equation relates to black hole entropy and how we can define it in relation to the original Hawking-Bekenstein black hole entropy formula. We can see below the original formula,

The equation above tells us the amount of entropy of a black hole. Originally Bekenstein derived this formula and concluded a black hole entropy is proportional to the black hole’s event horizon surface area.
The Fine Structure Constant
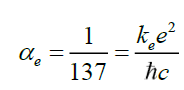
Above we have an equation which gives us the value of the fine structure constant. We represent the fine structure constant as 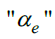 . The variable
. The variable 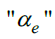 can also be given by the second expression
can also be given by the second expression 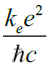 .This expression essentially implies that the fine structure constant is a probability which shows the chance of an electromagnetic interaction between a photon and an electron within an atomic electrostatic interaction. The probability is one out of 137 atomic electrostatic interactions emitting or absorbing a photon.
.This expression essentially implies that the fine structure constant is a probability which shows the chance of an electromagnetic interaction between a photon and an electron within an atomic electrostatic interaction. The probability is one out of 137 atomic electrostatic interactions emitting or absorbing a photon.
The New Micro-State Variable for Black Hole Entropy
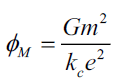
This is the micro-state variable we will use in our new black hole entropy equation. The variable tells us how many atomic electrostatic interactions are needed within a certain surface area to result in the gravitational field of the mass “m”. Keep in mind the surface area is derived using the Schwarzschild radius of the mass "m". Whenever the mass “m” equals the Planck mass,  equals which is the maximum of the fine structure constant [1].
equals which is the maximum of the fine structure constant [1].
Multiplying the fine structure constant and the micro-state variable,
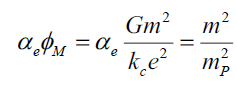
The product of both the fine structure constant αe and the micro-state variable  give us the squared number of Planck masses "mP" within an overall mass "m". Within each Planck mass there are 137 atomic electro-static interactions and each 137 electrostatic interaction there are at least one of the interactions has an electro-magnetic absorption or emission of a photo.
give us the squared number of Planck masses "mP" within an overall mass "m". Within each Planck mass there are 137 atomic electro-static interactions and each 137 electrostatic interaction there are at least one of the interactions has an electro-magnetic absorption or emission of a photo.
Presenting a New Form of Black Hole Entropy
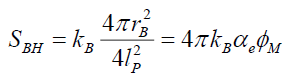
This is the black hole entropy equation in its original form reduced to 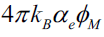 . The original Hawking-Bekenstein equation makes the black hole entropy proportional to the surface area
. The original Hawking-Bekenstein equation makes the black hole entropy proportional to the surface area  where "rB" is the Schwarzschild radius of the mass “m”."rB"equals 2Gm divided by c2.The new form makes
where "rB" is the Schwarzschild radius of the mass “m”."rB"equals 2Gm divided by c2.The new form makes 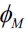 the micro-state variable proportional to the black hole entropy SBH.
the micro-state variable proportional to the black hole entropy SBH.
Keep in mind 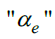 is the fine structure constant and
is the fine structure constant and 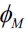 equals
equals 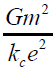 .
.
This new form of black hole entropy needs to be interpreted because of how I have defined above the variables of the fine structure constant and the micro-state [2].
I conclude black hole entropy in this new form can be interpreted as a measure of the different states in which a certain number of atomic electro-static interactions can be arranged in a Schwarzschild surface area to result in the gravitational field of mass “m”.
List of Physical Parameters
| SB | Black hole entropy |
| kB | Boltzmann constant, 1.3807×10-23JT-1 |
| c | Speed of light , 299792458 ms-1 |
| G | Gravitational constant, 6.674×10-11m3kg-1s-2 |
| m | Mass of black hole |
| rb | Schwarzschild radius of mass “m” |
| Ip | Planck length, 1.6162×10-35m |
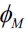 |
Micro-state variable of black hole entropy |
| mP | Planck mass, 2.176×10-8 kg |
REFERENCES
- Penrose Roger. Vintage books. 2005
- Alberto Coe. Micro Black Hole Entropy and Fine Structure Constant: A Numerological Approach. 2021

