Research

, Volume: 13( 6)

• View PDF
• Download PDF

Planck's Hubble constant and 120 Supernovae Type Ia show that the expansion of our local Universe is decelerating

*Correspondence:

Göran Henriksson
Department of Physics and Astronomy, Uppsala University, Uppsala, Sweden
E-mail: goran.henriksson@physics.uu.se

Abstract

The Bayesian regression curve for 120 Supernovae Type Ia, Khetan et al., 2021, corresponds within the margin of errors to the Hubble constant H₀=67.4 kms^-1 Mpc^-1 determined by the Planck Collaboration in 2020. If we from this curve read which distance modulus μ that corresponds to the redshift z=0.08 we get μ=37.76. From an equation by Stephen Weinberg, published in 1972, we can now calculate the cosmic deceleration q₀=+1.060 ± 0.039. This possibility was found during a successful test of the Hubble constant H₀=67.3 kms^-1 Mpc^-1, determined from the BL Lac object AP Lib, against the calibration by Khetan et al. This means that H₀, determined by the Planck Collaboration in 2020, and the well-calibrated sample of Supernovae Type Ia, by Khetan et al., proves that the expansion of our local Universe is decelerating at least within the nearest 1 billion light years. The q₀=+1.075 value, from AP Lib, gives an independent support to this result because it falls within the limits of error q₀=+1.060 ± 0.039 of Planck's final result.

Keywords

Supernovae Ia; Hubble constant; Deceleration of universe; Kerr black hole; Massive gravity

Introduction

Bengt Westerlund, former Director of ESO in Chile, 1969–1975, had performed photoelectric observations of the BL Lacerate object AP Lib 1969–1982 [1]. In 1984, he asked the author of the present paper to analyze his and published observations. Two periodic variations were discovered with periods, 953.806 and 45.357 days. A phase shift of the shorter period with amplitude ± 11.5 days is caused by orbital motion of a secondary object, with intrinsic periodic 45.357 days, around a primary object with the orbital period 953.806 days. The conclusion is that AP Lib is a binary black hole system with the possibility to determine the total mass from Kepler's third law. To calculate the absolute magnitude of the accretion disk around a Kerr black hole, a computer program was developed according to a theory by Kip Thorne [2]. To test the results the Hubble constant=H₀ was calculated. Results from the recent paper by Khetan et al. (2021, hereafter K21) seemed to offer a good possibility to test the quality of the H₀ value and distance modulus, μ=m-M, determined from AP Lib. (m=apparent magnitude and M=absolute magnitude.) The authors of this paper had performed a new calibration of the peak absolute magnitude of Type Ia Supernovae (SNe Ia) based on the Surface Brightness Fluctuations (SBF) method in order to measure the value of the Hubble constant [3]. They built a sample of calibrating anchors consisting of 24 SNe hosted in galaxies that have SBF distance measurements. They applied a hierarchical Bayesian approach, calibrated the SN Ia peak luminosity and extended the Hubble diagram into the Hubble flow by including a sample of 96 SNe Ia in the redshift range 0.02 < z < 0.075, which they extracted from the Combined Pantheon Sample (CPS). They estimated a value of H₀=70.50 ± 2.37 (stat.) ± 3.38 (sys.) kms^-1 Mpc^-1 (i.e., 3.4% stat., 4.8% sys.), which is in agreement with the value obtained using the tip of the red giant branch calibration [4].

The q₀=1.075 value was calculated in May 2022. Several recent papers indicate problems with the Standard λCDM-model. On 2023-09-11 Nhat-Minh Nguyen et al. published: Evidence for Suppression of Structure Growth in the Concordance Cosmological Model [5]. On 2024-02-10 Adam Riess et al. published a paper with the title: JWST Observations Reject Unrecognized Crowding of Cepheid Photometry as an Explanation for the Hubble Tension at 8σ Confidence [6]. Charlie Wood published on 2024-04-04 the paper: Dark Energy May Be Weakening, Major Astrophysics Study Finds [7].

Method

The investigation started with a search for periods in the photoelectric observations by the author's own computer program. In fact the best solutions were pairs of periods and it was impossible to choose the best pair. This problem was solved by a similar search for periods in a sample of photographic observations performed with the Metcalf 10 inch astrograph at the Harvard observatory during the 1930^th. There was only one common pair of periods in the two samples. When both samples was tested together the final periods were p=953.806 days and 45.357 days. In the residuals there was found a third period p=3.21755 days which corresponds to the motion in the last marginally stable orbit in the accretion disk around the central black hole. These periods can also be found in observations at radio wavelengths and from optical polarisation. The corresponding light curves were analyzed by a computer program constructed by the author. In these program periodic variations with up to three independent periods can be presented as Fourier curves with individually chosen order. The periodic variation with p=953.806 days can be descried as a seventh order Fourier curve with three max and min. The p=45.357 days variations corresponds to a third order Fourier curve with two max and min and the p=3.21755 days variation is approximated as a fourth order Fourier curve with three max and min. The three max and min of the 953.806 days curve are caused by eclipses of the central accretion disk by clouds at the Lagrange points in the binary system L3, L4 and L5. However, there is also a significant peak at the phase when the two black holes are aligned between L4 and L5, when the smaller black hole acts as a gravitational lens for the brightest spot in the accretion disk around the central black hole. At that phase the optical polarisation is heavily disturbed. Calculations of eclipses of the central accretion disk by the three clouds show that the highest angular altitude of the clouds from the orbital plane is 5º ± 1º. The motion of the three objects in the last marginally stable orbit with p=3.21755 days is best studied at radio wavelength. AP Lib is a binary black hole system with the inclination of the orbital plane of 87º ± 1º. Between L3, L4 and L5, there exist gaps that make it possible to directly see the accretion disk around the central massive black hole. The accretion disk is not homogenous because the infilling matter can only enter the disk in the gaps between L3, L4 and L5 and in the innermost orbit only Doppler boosted light from one of the mass concentrations can be directed towards the observer at the same time. The total mass of the system, calculated from the third law by Kepler is 5% greater than the mass of the primary object calculated from the orbital period of the last marginally stable orbit at the inner edge of the accretion disk. In the analysis of the photometry of AP Lib, the contribution from the two objects had to be separated to make it possible to study them individually and to subtract scattered light in the system [1]. Careful removal of the scattered light was necessary to isolate the light from the accretion disk around the central black hole and to determine its apparent magnitude (m). The mass of the central black hole is 1.3 × 109 M (sun). The absolute magnitude (M) of the accretion disk was calculated using Kip Thorne's Model [2]. The light emitted from the accretion disk towards the observer must be corrected for its inclination, the mass and spin of the central black hole, the energy efficiency factor, and the light extinction. To check if the model and the computer program worked correctly, the author decided to calculate the Hubble constant H0 [8].

To investigate the deceleration of the Universe the author used a purely kinematic cosmological model that gives the luminosity distance as a function of redshift Weinberg and Visser [9, 10].The parameterization assumes a Robertson–Walker metric in a flat space for the geometry of the Universe and it is based on the Taylor series expansion of the Hubble–Lemaȋtre law. This law is expressed using two additional parameters, q₀ and j₀, where q₀ is the cosmic deceleration and j₀ is the third derivative of the scale factor, the so-called cosmic jerk K21 [3]. Assumed that the Universe is flat and limited their calculations to the expansion of the luminosity distance to the second order in z, the redshift. If one neglects O (z³) and higher-order terms, the corresponding distance modulus is given as:

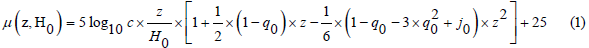
where c is the speed of light.

They fixed the value of the deceleration and jerk parameters to q₀=-0.55 and j₀=1, [11, 12]. In their redshift range of cosmological sample (0.009 <z<0.075), K21 assumed that any assumption about the expansion history of the Universe does not significantly affect the final estimate of H0 and they concluded that fixing the values of q0 and j0 does not bias their estimates of H₀ [13].

Results

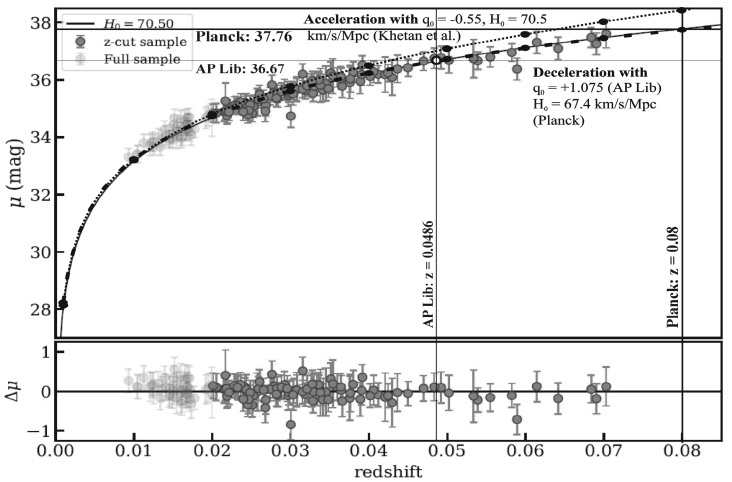
From the investigation of AP Lib it was found that H₀=67.3 kms^-1 Mpc^-1 and the distance modulus m-M=36.67 [8]. The author's value of H₀=67.3 kms^-1 Mpc^-1 is determined using the datum of AP Lib, and should if valid apply to all points on the regression curve according to Eq. (1) [9, 10]. From Eq. (1), it was now possible to calculate the deceleration q₀ from μ, z and H₀, with j₀=1. With H₀=67.3 kms^-1 Mpc^-1 and the distance modulus μ=36.67 (determined from AP Lib) and its redshift z=0.0486, and j₀=1, we get q₀=1.075, which is significantly positive. From the regression curve K21, we can read the value
μ=37.76 for the redshift z=0.080 and with H₀=67.4 ± 0.5 kms^-1 Mpc^-1, the final result of the Planck project, we can calculate q₀=1.060 ± 0.039, see the thick solid lines in FIG. 1. These values imply that the expansion of our local Universe is slowing down. In the last twenty years different research groups have proposed values for the Hubble constant, 70<H₀<76 kms^-1 Mpc^-1. However, the final result from the Planck project is H₀=67.4 ± 0.5 kms^-1 Mpc^-1 and it differs not significantly from the authors value H₀=67.3 kms^-1 Mpc^-1. On the other hand, these two values differ significantly from the values of H₀ determined from the most recent analyses of Supernovae. This is a great problem in cosmology because the difference is of the order 5 sigma [12-14].

Discussion

K21 claim that their H₀=70.5 kms^-1 Mpc^-1 corresponds to the curve in their figure. However, K21 estimated the Hubble constant H₀ from eq. (1) in the present paper. The scrutiny of the relevance of the AP Lib results began by plotting the value of the distance module and the measured redshift of Disney et al. [14]. A top the results of K21 that figure shows their calibrated distance module versus the redshift and the corresponding Bayesian regression curve. It is an unexpected outcome that the AP Lib datum falls exactly atop the regression curve.

However, this perfect match confirms the relevance of the calibration of the observations and the quality of the theoretical model for the radiation from an accretion disk around a Kerr black hole by Thorne [2]. It is important that this completely independently determined value is consistent with the other values in the figure, which are based on photometric calibrations of Supernovae. However, the Supernovae values are somewhat scattered about the regression curve.

To calculate H₀, K21 used q₀=−0.55 and j₀=1 and Eq. (1) to get H₀=70.5 5 kms^-1 Mpc^-1. In their figure, they plotted the distance modulus from their cosmological samples versus redshift [3]. The distance modulus is computed from the luminosity calibration relation obtained with the SBF sample. The solid curve in the same figure shows the best-fit model derived from a Bayesian regression and the residuals are shown in the bottom panel. The author calculated a curve that corresponds to their values q₀=−0.55, j₀=1 and H₀=70.5 kms^-1 Mpc^-1 and over plotted this curve on their FIG. 1 as the dotted curve with the calculated black dots.

A corresponding curve for a solution consisting of q₀=1.075, from AP Lib, and H₀=67.4 kms^-1 Mpc^-1, from CMB by the Planck collaboration was calculated [12]. This is displayed as the dashed curve with black dots in FIG. 1. It is worth noting that in FIG. 1, the black regression curve determined by K21 falls under the dashed curve. This means that this solution agrees perfectly in all details with the Bayesian regression curve that represents the calibrated data by K21. These data can be used to determine the deceleration q₀=+1.060 ± 0.039 of our local Universe at least within distances of 1 billion light years.

Summary

Initially, the main reason to write this paper was to test the quality of the results from the analysis of the binary black hole system AP Lib and especially the value of H₀=67.3 kms^-1 Mpc^-1 and the distance modulus m-M=36.67 by Henriksson and the observed z=0.0486 [14]. K21seemed to be a very good choice for this test. The value from AP Lib, the black circle with white filling, falls perfectly on the regression curve in FIG. 1. However, the conclusion that the local Universe is decelerating is the main result of this paper. The validity is strongly supported by the fact that the value of the Hubble constant H₀=67.3 kms^-1 Mpc^-1, determined from observations of AP Lib, differs insignificantly from the final result 67.4 kms^-1 Mpc^-1 of Planck collaboration . To avoid subjective misinterpretations when different authors opinions are confronted the present author has chosen to use exact quotations of their own texts and use definitions from The SAO Encyclopaedia of Astronomy [3-15].

Giostri et al. present results from an analysis that includes higher order than two in z. They write in their abstract:

We use Type Ia Supernovae (SN Ia) data in combination with recent Baryonic Acoustic Oscillations (BAO) and Cosmic Microwave Background (CMB) observations to constrain a kink-like parameterization of the deceleration parameter (q). This q-parameterization can be written in terms of the initial (q_i) and present (q₀) values of the deceleration parameter, the redshift of the cosmic transition from deceleration to acceleration (z_t) and the redshift width of such transition (τ). Our results indicate, with a quite general and model independent approach, that MLCS2k2 (Jha et al. 2007) favors Dvali Gabadadze Porrati like cosmological models, while SALT2 (Guy et al. 2007) favors λCDM-like ones. Progress in determining the transition redshift and/or the present value of the deceleration parameter depends crucially on solving the issue of the difference obtained when using these two light-curve fitters. The references correspond to the intense search for distant Supernovae resulted in the two important papers of Riess et al. and Perlmutter et al. In both papers, "Dark Energy" plays an important role. According to The SAO Encyclopaedia of Astronomy: Dark Energy is a hypothetical form of energy that exerts a negative, repulsive pressure behaving like the opposite of gravity. It has been hypothesised to account for the observational properties of distant Type Ia Supernovae, which show the Universe going through an accelerated period of expansion [16-19].

According to SAO, both research teams announced that:

Distant, z=1 Type Ia Supernovae were slightly too faint than model predictions of an expanding (yet slowing) Universe. To be fainter, the Supernovae must be farther away and this requires that the expansion of the Universe was slower in the past. Both teams agreed that the Universe is going through a phase of accelerated expansion. Dark Energy was invoked to drive this acceleration.

However, the observations by K21 shown in FIG. 1, fit perfectly with a Universe that is decelerating within at least the nearest 1 billion light years. Because the Dark Energy is described as a homogenous accelerating field, opposite of gravity, distributed throughout the Universe, our local decelerating volume should not exist. The result of this paper can be interpreted as a proof against the existence of the hypothetical Dark Energy. It should be pointed out that the well-established Eq. (1) is not good enough for z>0.24. It is necessary to introduce higher orders than two in z to determine q₀ for z=1 and the result is no longer unique.

On the other hand, the model by Dvali et al. is based on Massive Gravity. They have included the mass of the gravitational field in their solution of Einstein's equations. The author has tested this theory on a local scale such as our solar system by studying the lunar secular acceleration back to 3653 BC from identifications of 33 total solar eclipses Henriksson. When all known components of the lunar secular acceleration were taken into account, including the precession of the geodesic by Einstein, there was still a significant residual effect that matched the theory of Massive Gravity [20-23].

The study began with the determination of the radius of crossover when the Universe had become so big that not all gravitons could reach the most distant galaxies and the gravitational forces between these galaxies weakened. This has been interpreted as an acceleration of the Universe. From the radius of crossover it was possible to calculate the mass of the graviton because the radius of crossover is defined as the inverse of the mass of the graviton. This acceleration started 4.54 Gy after the Big Bang and the mass of the graviton m_g=1.31 ± 0.01∙10⁻⁵⁶ g Henriksson. However, this predicted acceleration of the Universe has no effect in our local volume of the Universe and the deceleration determined in this paper is not in conflict with the theory of Massive Gravity [21-23].

Conclusions

It is remarkable that the value of the Hubble constant seems to have been constant during the evolution of the Universe since the emission of the CMB radiation. The expansion of the Universe is decelerating in our local Universe. However, this local deceleration is not in conflict with the acceleration of the Universe at great distances predicted in the theory of Massive Gravity. The author's analysis shows that there is no need for Dark Energy.

Acknowledgements

I acknowledge useful discussions with my colleagues at the Astronomical Observatory in Uppsala: PhD's Ola Karlsson, Christer Sandin and Kjell Lundgren.

Declaration of Competing Interests

There exist no competing interests.

Funding Sources

This research did not receive any grant from funding agencies in public, commercial, or not for profit sectors.

References

1. Westerlund BE, Wlerick G, Garnier R. The properties of AP Librae from UBV photoelectric photometry. Astron Astrophys. 1982;105:284-92.

   [Google Scholar]

2. Thorne KS. Disk-accretion onto a black hole. II. Evolution of the hole. Astrophys J.1974; 191:507-20.

   [Google Scholar]

3. Khetan N, Izzo L, Branchesi M, et al. A new measurement of the Hubble constant using Type Ia supernovae calibrated with surface brightness fluctuations. Astron Astrophys. 2021;1:647-72.

   [Google Scholar] [Crossref]

4. Freedman WL, Madore BF, Hoyt T, et al. Calibration of the tip of the red giant branch. Astrophys J.2020 4;891(1):57.

   [Google Scholar]

5. Nguyen NM, Huterer D, Wen Y. Evidence for suppression of structure growth in the concordance cosmological model. Phys Rev Lett. 2023;15;131(11):111-01.

   [Google Scholar] [Crossref]

6. Riess AG, Anand GS, Yuan W, et al. JWST Observations Reject Unrecognized Crowding of Cepheid Photometry as an Explanation for the Hubble Tension at 8σ Confidence. Astrophys J Lett. 2024;6;962(1):17.

   [Google Scholar] [Crossref]

7. Wood, C., 2024. Dark Energy May Be Weakening, Major Astrophysics Study Finds, Quanta Magazine, April 4, 2024. .[Google Scholar] [Crossref]
8. Henriksson G. A Relativistic Binary Black Hole Model for AP Lib: with Kip Thorne's Kerr Black Hole. Lambert Academic Publishing; 2018.

   [Google Scholar]

9. Weinberg S. Gravitation and Cosmology:Principles and Applications of the General Theory of Relativity. Inc.

   [Google Scholar]

10.  Visser M. Jerk, snap and the cosmological equation of state. Class Quantum Gravity. 2004;21(11):2603.

    [Google Scholar] [Crossref]

11. Betoule ME, Kessler R, Guy J, et al. Improved cosmological constraints from a joint analysis of the SDSS-II and SNLS supernova samples. Astron Astrophys. 2014;568: 22.

    [Google Scholar] [Crossref]

12. Collaboration P, Aghanim N, Akrami Y, et al. Planck 2018 results. VI. Cosmological parameters.

    [Google Scholar] [Crossref]

13. Dhawan S, Brout D, Scolnic D, et al. Cosmological model insensitivity of local H0 from the Cepheid distance ladder. Astrophys J. 2020;894(1):54.

    [Google Scholar]

14. Disney MJ, Peterson BA, Rodgers AW. The redshift and composite nature of AP Librae/PKS 1514-24. Astrophys J.194, Dec. 1, 1974, pt. 2, p. L79-L82.. 1974 Dec;194:L79-82.

    [Google Scholar]

15. Giostri R, dos Santos MV, Waga I, et al. From cosmic deceleration to acceleration: new constraints from SN Ia and BAO/CMB. J Cosmol Astropart Phys. 2012;15(03):027.

    [Google Scholar] [Crossref]

16. Jha S, Riess AG, Kirshner RP. Improved distances to type Ia supernovae with multicolor light-curve shapes: MLCS2k2. The Astrophysical Journal. 2007;659(1):122.

    [Google Scholar] [Crossref]

17. Guy J, Astier P, Baumont S, et al. SALT2: using distant supernovae to improve the use of type Ia supernovae as distance indicators. Astron Astrophys. 2007;1;466(1):11-21.

    [Google Scholar] [Crossref]

18. Riess AG, Filippenko AV, Challis P, et al. Observational evidence from supernovae for an accelerating Universe and a cosmological constant. astron j.1998;116(3):1009.

    [Google Scholar] [Crossref]

19. Perlmutter S, Aldering G, Goldhaber G, et al. Measurements of Ω and Λ from 42 high-redshift supernovae. Astrophys J. 1999;517(2):565.

    [Google Scholar] [Crossref]

20. Dvali G, Gabadadze G, Porrati M. 4D gravity on a brane in 5D Minkowski space. Phys Lett  B. 2000;485(1-3):208-14.

    [Google Scholar] [Crossref]

21. Dvali G, Gruzinov A, Zaldarriaga M. The accelerated Universe and the Moon. Phys Rev D. 2003;68(2):024012.

    [Google Scholar] [Crossref]

22. Henriksson G. The acceleration of the Moon and the Universe. In51th rencontres de moriond 2016 cosmol. 2016;51:259-60. ARISF.

    [Google Scholar]

23. Henriksson G. The acceleration of the Moon and the Universe-the Mass of the Graviton. Adv Astrophys. 2017;2(3):184-96.

    [Google Scholar] [Crossref]